1 J
Peter has a gold bar of size 2 \times 3 \times 4. Being an amateur blacksmith, he melted down the bar and created three identical cubes from the liquid gold. What is the common sidelength of Peter’s cubes?
2
2 J
A new Year’s Eve celebration was attended by 43 people. The bar was selling juice, beer, and champagne. During the night 25 people drank beer, 19 people drank champagne, and 12 people drank both beer and champagne. The others were drivers, so they drank only juice. How many people drank only juice?
11
3 J
By drinking a cup of black tea, one gets enough caffeine for one hour. By drinking a cup of coffee, one gets caffeine for 4 hours. In what ratio should we mix black tea and coffee in order to have a full cup containing caffeine for two hours?
2:1
4 J
The mirrors v and h in the picture are perpendicular to each other. The angle between the mirrors h and s is 75^\circ. A light ray was incident on the mirror v, from which it reflected to h, then to s, and finally back to v again. The initial angle of incidence on v was 50\stupen. What was the angle of incidence on v after reflecting from s?
Note: The angle of reflection equals the angle of incidence.
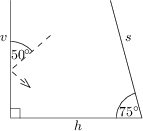
80^\circ
5 J
A shop sold 235 intergalactic spaceships during the year 2013. In each month, 20, 16, or 25 spaceships were sold. Determine in how many months the shop sold exactly 20, 16, and 25 spaceships, respectively.
4, 5, 3
6 J
Two circles have radii 5 and 26. The centre of the larger circle lies on the circumference of the smaller one. Consider the longest and the shortest chord of the larger circle which are tangent to the smaller circle. What is the difference between the lengths of these extremal chords?
4
7 J
Collin Farrel was born in the last century in Sleepy Hollow. We know that his age in 1999 was the same as the sum of the digits of the year when he was born. What is Collin’s year of birth?
1976
8 J
Four circles of radius 1 are pairwise tangent (except for the top and the bottom one) as in the figure below. A tight band is wrapped around them, as shown in the figure. What is the length of the band?
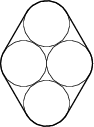
8+2\pi
9 J
A certain sports teacher always orders his class of bugs by the number of their legs. In the last sports session, only Buggy and four other bugs, who have 6, 3, 10, and 9 legs, were present. We know that when they stood in the correct order, the number of legs of the middle bug was the arithmetic mean of the numbers of legs of all five bugs. Find all possible numbers of Buggy’s legs.
2, 7, 17
10 J
For non-zero digits X and Y, denote \overline{XY} the number whose decimal representation is XY. Assuming non-zero digits A, B, C satisfy \overline{AA} + \overline{BB} + \overline{CC} = \overline{ABC}, determine \overline{ABC}.
198
11 J / 1 S
The famous celestial object collector Buggo was forced to sell one third of his collection due to the financial crisis. Immediately afterwards he gave three of his Solar System planets to his daughter. Later, he sold one third of the rest of his collection, and, furthermore, he also gave two moons of Jupiter and two moons of Saturn to his wife. Finally he sold one third of the rest of his objects followed by Mars with both its moons, and after that there were only 9 planets in the Alpha Centauri system left in his collection. How many objects did Buggo have at the beginning?
54
12 J / 2 S
Find the least positive integer greater than 2014 that cannot be written as a sum of two palindromes.
Note: A palindrome is a number whose digits (in decimal representation) are the same read backward.
2019
13 J / 3 S
Vodka gave Ondro a number puzzle. He chose a digit X and said: “I am thinking of a three digit number that is divisible by 11. The hundreds digit is X and the tens digit is 3. Find the units digit.” Ondro soon realised that he had been cheated and there is no such number. Which digit X did Vodka choose?
4
14 J / 4 S
The real numbers a, b, c, d satisfy a < b < c < d. If we pick all six possible pairs of these numbers and compute their sums, we obtain six distinct numbers, the smallest four being 1, 2, 3, and 4. Determine all possible values of d.
3.5, 4
15 J / 5 S
Jack is twice as old as Jill was when Jack was as old as Jill is today. When Jill is as old as Jack is now, the sum of their ages will be 90. How old is Jack now?
40
16 J / 6 S
Positive integers a_1 , a_2 , a_3, \ldots form an arithmetic progression. If a_1 = 10 and a_{a_2} = 100, find a_{a_{a_3}}.
820
17 J / 7 S
Natali’s favorite number has the following properties:
- it has 8 digits;
- its digits are pairwise distinct and decreasing when read from left to right; and
- it is divisible by 180.
What is Natali’s favorite number?
97654320
18 J / 8 S
Kika likes to build models of three-dimensional objects from square ruled paper. Last time she scissored out a shape as shown in the figure below. Then she glued it together in such a way that no two squares were overlapping, there were no holes in the surface of the resultant object and it had nonzero volume. How many vertices did this object have? Note that by a vertex we mean a vertex of the three-dimensional object, not a lattice point on the paper.
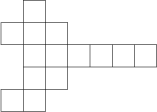
12
19 J / 9 S
Find all pairs of positive integers a, b such that ab = \gcd(a,b) + \lcm(a,b), where \gcd(a,b) and \lcm(a,b) are the greatest common divisor and the least common multiple of a and b, respectively.
(2, 2)
20 J / 10 S
There are 29 unit squares in the diagram below. A frog starts in one of the five (unit) squares in the bottom row. Each second, it jumps either to the square directly above its current position (if such a square exists), or to the square that is one above and one to the right from its current square (if such a square exists). The frog jumps every second until it reaches the top. How many distinct paths from the bottom row to the top row can the frog take?
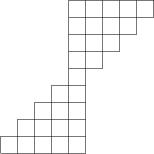
256
21 J / 11 S
Palo is walking along the diagonals of a regular octagon. His walk begins in a fixed vertex of the octagon, and should continue along all the diagonals in such a way that he walks along each diagonal exactly once. How many different walks can Palo take?
0
22 J / 12 S
Consider a 2014 \times 2014 grid of unit squares with the lower left corner in the point (0,0) and upper right corner in (2014,2014). A line p passes through the points (0,0) and (2014,2019). How many squares of the grid does p cross?
Note: A line crosses a square if they have at least two points in common. For example, the diagonal crosses 2014 squares of the grid.
4023
23 J / 13 S
A convex n-gon has one interior angle of an arbitrary measure and all the remaining n-1 angles have a measure of 150^\circ. What are the possible values of n? List all possibilities.
8, 9, 10, 11, 12
24 J / 14 S
If the sides a, b, c of a triangle satisfy what is the angle between the sides b and c?
60^{\circ}
25 J / 15 S
Find all integers between 1 and 200, whose distinct prime divisors sum up to 16. (For example, the sum of the distinct prime divisors of 12 is 2 + 3 = 5.)
66, 132, 198, 55, 39, 117
26 J / 16 S
The lengths of line segments in the figure satisfy DA = AB = BE, GA = AC = CF and IC = CB = BH. Moreover, EF = DI = 5 and GH = 6. What is the area of triangle ABC?
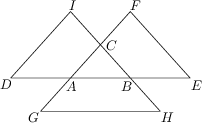
3
27 J / 17 S
We call a prime p strong if one of the following conditions holds:
- p is a one-digit prime; or
- if we remove its first digit, we obtain another strong prime, and the same holds for the last digit.
For example, 37 is a strong prime, since by removing its first digit we get 7 and by removing its last digit we get 3 and both 3 and 7 are strong primes. Find all strong primes.
2, 3, 5, 7, 23, 37, 53, 73, 373
28 J / 18 S
Let k and l be two circles of radius 16 such that each of them passes through the centre of the other. A circle m is internally tangent to both k and l and also tangent to the line p passing through their centres. Find the radius of the circle m.

6
29 J / 19 S
How many six-digit positive integers satisfy that each of their digits occurs the same number of times as is the value of the digit? An example of such an integer is 133232.
82
30 J / 20 S
True to his reputation as a cool guy, E.T. glued together 4 balls of radius 1 so that they were pairwise tangent. What is the radius of the smallest sphere containing E.T.’s 4 balls?

1 + \frac{\sqrt{6}}{2}
31 J / 21 S
Determine the number of pairs of positive integers (x, y) with y < x \leq 100 such that the numbers x^2 - y^2 and x^3 - y^3 are coprime.
99
32 J / 22 S
Consider a number that starts with 122333444455555 \ldots and continues in such a way that we write each positive integer as many times as its value indicates. We stop after writing 2014 digits. What is the last digit of this number?
4
33 J / 23 S
What is the smallest positive integer N for which the equation (x^2 - 1)(y^2 - 1) = N has at least two distinct integer solutions (x,y) such that 0<x\leq y?
360
34 J / 24 S
Let two circles of radii 1 and 3 be tangent at point A and tangent to a common straight line (not passing through A) at points B and C. Find AB^2+BC^2+AC^2.
24
35 J / 25 S
Find the largest prime p such that p^p \mid 2014!.
43
36 J / 26 S
Consider an array of 2 columns and 2014 rows. Using 3 different colours, we paint each cell of the array with a colour so that if the cells share a wall, they are of different colours. How many different colourings are there?
6 \cdot 3^{2013}
37 J / 27 S
Find the smallest positive integer m such that 5m is the fifth power of an integer, 6m is the sixth power of an integer and 7m is the seventh power of an integer.
5^{84}6^{35}7^{90}
38 J / 28 S
We fold a rectangular piece of paper in such a way that two diagonally opposite vertices are touching, thus creating a fold, i.e. a line segment across the paper. After folding, the length of the fold line has the same length as the longer side of the rectangle. What is the ratio of the length of a longer side to the length of a shorter side of the rectangle?
\sqrt{\frac{1 + \sqrt{5}}{2}}
39 J / 29 S
We have 20 marbles, each of which is either yellow, blue, green, or red. Assuming marbles of the same colour are indistinguishable, how many marbles can be blue at most if the number of ways we can arrange the marbles into a straight line at most is 1140?
17
40 J / 30 S
Find all integers n\ge 3 such that the regular n-gon can be divided into at least two regular polygons.
3, 4, 6, 12
41 J / 31 S
A rook is standing in the bottom left corner of a 5\times 5 chessboard. In how many ways can the rook reach the top right corner of the board, provided that it is limited to move only up or to the right? We consider two paths to be distinct if the sequences of the visited squares are different.
838
42 J / 32 S
What is the digit in the place of hundreds in 11^{2014}?
2
43 J / 33 S
A grasshopper is jumping on vertices of an equilateral triangle. Whenever it sits on a vertex, it randomly chooses one of the other two vertices as the destination for its next jump. What is the probability that it returns to the starting vertex with its tenth jump?
\frac{171}{512}
44 J / 34 S
Parsley is a lumberjack. His work is strictly divided into minutes, in which he may choose one of the following actions:
- He cuts down n trees, where n is his current power. This wears him out, so his power decreases by one.
- He takes a rest and restores one point of power.
What is the maximal number of trees he may cut down in 60 minutes, if his initial power is 100?
4293
45 J / 35 S
Denote by \sqrt{\mathbb N} the set \{\sqrt{1}, \sqrt{2}, \sqrt{3}, \sqrt{4}, \dots \}, i.e. the set of all square roots of positive integers. Let S be the set of real numbers a for which both a \in \sqrt{\mathbb{N}} and 36/a \in \sqrt{\mathbb{N}} hold. What is the product of the elements of the set S?
6^{25}
46 J / 36 S
What is the probability that the product of 2014 randomly chosen digits is a multiple of 10?
1-\bigl(\frac{5}{10}\bigr)^{2014} - \bigl(\frac{8}{10}\bigr)^{2014} + \bigl(\frac{4}{10}\bigr)^{2014}
47 J / 37 S
Evaluate the expression Note: The symbol \lfloor x \rfloor denotes the integral part of x, i.e. the greatest integer not exceeding x.
-2002
48 J / 38 S
Boris counted the sum 1+2+\cdots +2012. However, he forgot to add some numbers and got a number divisible by 2011. Ann counted the sum A = 1+2+\cdots +2013. However, she missed the same numbers as Boris and got a number N divisible by 2014. What is the ratio N/A?
2/3
49 J / 39 S
A game is played with 16 cards laid in a row. Each card has one side black and the other side red. A move consists of taking a consecutive sequence of cards (possibly only containing 1 card) in which the leftmost card has its black side face-up and the rest of the cards have red side face-up, and flipping all of these cards over. The game ends when a move can no longer be made. What is the maximum possible number of moves that can be made before the game ends?
2^{16} - 1
50 J / 40 S
We have a right-angled triangle with sides of integral lengths such that one of the catheti is 2014^{14} in length. How many such triangles exist up to congruence?
\frac{27 \cdot 29^2 - 1}{2}
51 J / 41 S
What is the smallest positive integer that cannot be written as a sum of 11 or fewer (not necessarily distinct) factorials?
359
52 J / 42 S
In a chess tournament, each participant plays against every other participant, and there are no draws. Call a group of four chess players ordered if there is a clear winner and a clear loser, that is, one person who beat the other three and one person who lost to the other three. Find the smallest integer n for which any chess tournament with n people has a group of four chess players that is ordered.
8
53 J / 43 S
I chose two numbers from the set \{1,2,\ldots, 9\}. Then I told Peter their product and Dominic their sum. The following conversation ensued:
- Peter: “I don’t know the numbers.”
- Dominic: “I don’t know the numbers.”
- Peter: “I don’t know the numbers.”
- Dominic: “I don’t know the numbers.”
- Peter: “I don’t know the numbers.”
- Dominic: “I don’t know the numbers.”
- Peter: “I don’t know the numbers.”
- Dominic: “I don’t know the numbers.”
- Peter: “Now I know the numbers.”
What numbers did I choose?
2, 8
54 J / 44 S
Three identical cones are placed in the space in such a way that their bases are pairwise incident and all three bases completely lie in a single plane. We place a sphere between the cones so that the top of the sphere is in the same height as the vertices of the cones. What is the radius of the ball if each cone has base of radius 50 cm and height 120 cm?

\frac{200\sqrt{3}}{9}
55 J / 45 S
Imagine a rabbit hutch formed by 7 \times 7 cell grid. In how many ways can we accommodate 8 indistinguishable grumpy rabbits in such a fashion that every two rabbits are at least either 3 columns or 3 rows apart?
51
56 J / 46 S
Given noncollinear points A, B, C, the segment AB is trisected by points D and E. Furthermore, F is the midpoint of the segment AC, and EF and BF intersect CD at G and H, respectively. Compute [FGH] provided that [DEG] = 18. Note that by [XYZ] we denote the area of \triangle XYZ.
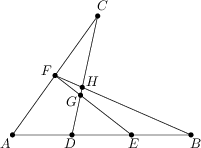
\frac{9}{5}
57 J / 47 S
The surface of a solid consists of two equilateral triangles with sides of length 1 and of six isosceles triangles with legs of length x and the base of length 1, as shown below. What is the value of x given that the volume of the object is 6?
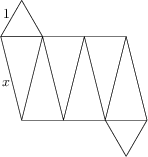
\frac{5\sqrt{39}}{3}