1 J
Given is a cubical-shaped boulder with an original volume of 216\,\text{m}^3. What size is the surface of the boulder in \text{m}^2 after knocking out a cuboidal block of dimensions 1\,\text{m}\times 1\,\text{m}\times 2\,\text{m} as shown in the picture below?

216
2 J
The two friends Christoph and Jonas hit the jackpot and bought a nice rectangular property of dimensions 35\,\text{m} by 25\,\text{m}. They are planning to build a twin house and share garden G of size 300\, \text{m}^2. The building floor plan can be seen in the picture:
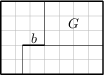
(The distance between two neighboring grid lines is 5\,\text m.) How far must wall b intrude from one section of the twin house into the other one so that the base areas of the friends’ parts are equal?
8.75\, \text{m}
3 J
Little Marcus wants to go to the beach. He owns the following distinguishable beach-outfits: 5 swimming trunks, 3 straw hats, 4 sunglasses and 5 T-shirts. To comply with the beach rules, he has to wear swimming trunks. Wearing sunglasses, hats and T-shirts is not obligatory at all, but if he puts on some outfit, he always takes at most one of each category. How many different ways are there for Marcus to appear in an appropriate outfit?
600
4 J
Laura spent her vacation in a rain forest. Each day it either rained in the morning, or it rained in the afternoon, or it rained the whole day. Laura enjoyed altogether 13 days, when it did not rain all the time, but experienced exactly 11 morning rains and 12 afternoon rains. How long was Laura’s vacation?
18 days
5 J
Find the smallest non-negative integer solution of the equation n-2\cdot \operatorname{Q}(n)=2016, where \operatorname{Q}(n) is the sum of the digits of n.
2034
6 J
How many positive integers have the property that their first (i.e. leftmost) digit is equal to their number of digits?
111\,111\,111
7 J
A paving consists of many pavers, one of which has the shape of a regular n-gon, completely surrounded by other pavers. When this paver is rotated by 48^\circ about its center, it fits again in its former position. What is the minimal n for which this is possible?
15
8 J
A day is called happy if its date written in the format DD.MM.YYYY consists of eight distinct digits—here DD fills in for the day, MM for the month and YYYY for the year, and if the day and the month is less than 10, a leading zero is prepended. For example, 26.04.1785 was a happy day. When is the next happy day (from now) going to be?
17.06.2345
9 J
How many different planes contain exactly four vertices of a given cuboid?
12
10 J
Little Sandra wants to draw a beautiful crescent using ruler and compass. First of all, she draws a circle with center M_1 and radius r_1=3\ \text{cm}. Then she sets the compass at a point M_2 of this circle and draws a second circle with radius r_2 which meets the first circle in antipodal points of a diameter through M_1, as shown in the picture below.
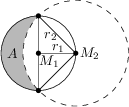
What is the area of the crescent A in \text{cm}^2?
9
11 J / 11 S
All servants of King Octopus have six, seven, or eight legs. The ones having seven legs always lie, whereas the ones having six or eight legs always tell the truth. One day, King Octopus assembled four of his servants and asked them how many legs the four of them had altogether. The first servant reported that the total number of legs was 25, the next one claimed 26, the third one said 27 and the last one 28. How many legs do the the king’s truth-telling servants (among these four) have in total?
6
12 J / 12 S
A shop sells bars of milk, white, and dark chocolate for the same price. One day, the shop earned 270 for the sold milk chocolate, 189 for the white chocolate, and 216 for the dark chocolate. What is the smallest total number of chocolate bars the shop could have sold on that day?
25
13 J / 13 S
A father of five children wants to have pastries for his family for tea time. Based on painful experience he knows that he has to distribute either the same type or five different types of pastry to his children, or else all kinds of heavy dispute will arise among the kids. One day, after a long discussion without any consent on the type of pastries, he exasperatedly instructed his youngest daughter Anna: “You’ll go to the pastry shop and ask the salesgirl to give you x pieces of pastries randomly! After you return home, each of the children shall get exactly one piece of pastry and the remaining pieces will be for mom and dad!” Assuming that the shop sells more than five types of pastry and it is always well stocked with every type, what number x did the father choose in order to keep the peace among his children in any case and to keep the costs as low as possible at the same time?
17
14 J / 14 S
What is the ratio of the area of a circle to the area of a square, perimeters of which are equal?
4 : \pi
15 J / 15 S
In February, Paul decided to visit the Cocos Islands with his private jet. He took off from his mansion in Europe at 10:00 Central European Time (CET) and landed on the Islands the next day at 5:30 local time (Cocos Islands Time, CCT). When returning home, he started at 8:30 CCT and landed at 17:00 CET the same day. Assuming that the duration of the flight was the same in both cases, what was the time on the Cocos Islands when Paul returned home?
22:30
16 J / 16 S
The numbers 14, 20, and n fulfill the following condition: Whenever we multiply any two of them, the result is divisible by the third one. Find all positive integers n for which this property holds.
70, 140, 280
17 J / 17 S
A rectangle is divided into two trapezoids along the line segment x as in the picture below. The distance PA is 10\,\text{cm} and AQ is 8\,\text{cm}. The area of the trapezoid T_1 is 90\,\text{cm}^2 and the area of T_2 is 180\,\text{cm}^2.
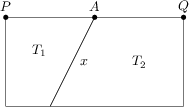
What is the length of the segment x in \text{cm}?
17
18 J / 18 S
Elisabeth has harvested strawberries in her garden. She wants to distribute them to her four sons in such a way that each son gets at least three strawberries and Valentin receives more strawberries than Benedikt, Benedikt more than Ferdinand, and Ferdinand more than Michael. Each son knows his number of strawberries, the total number of strawberries distributed, and the above-mentioned conditions. How should Elisabeth distribute the strawberries in order to hand as few as possible of them and none of her sons is able to determine the whole distribution?
(M,\, F,\, B,\, V) = (3,\, 5,\, 6,\, 8)
19 J / 19 S
We write all the integers from 1 to 1000 consecutively clockwise along the circumference of a circle. We now mark some of the numbers: Starting with 1, go clockwise and mark every 15th number (i.e. 16, 31 etc.). We continue this way, until we are forced to mark a number which we have already marked. How many numbers stay unmarked at the end of the procedure?
800
20 J / 20 S
Find the sum of the seven marked interior angles of this 7-pointed star (in degrees)!
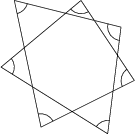
540^\circ
21 J / 21 S
Pupils were given the following exercise: They should compute the arithmetic mean of the numbers 1, 3, 6, 7, 8, and 10. However, Lucy chose a wrong approach: First she picked two of the numbers and computed their arithmetic mean. Then she computed the arithmetic mean of the result and some other number and repeated this step until she had used all the numbers. What is the largest absolute value of the error (i.e. the difference with the correct result) Lucy could have achieved?
17/6
22 J / 22 S
Along one side of a straight road there are five street lights L_1, L_2, L_3, L_4, and L_5 lined up equally spaced 12\, \text{m} apart. On the other side of the road there is an ice cream shop. If Julien is standing at the entrance E of the shop, the angle subtended at this point by L_1 and L_2 is \alpha=27^\circ. If he is standing at L_5, the angle at that point subtended by L_1 and E is 27^\circ, too.
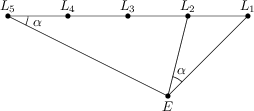
What is the distance from L_1 to E?
24\, \text{m}
23 J / 23 S
Clara chose two distinct integers from 1 to 17, inclusive, and multiplied them. Surprisingly, the product turned out to be equal to the sum of the remaining fifteen numbers. Find Clara’s two numbers.
10 and 13
24 J / 24 S
How many 6-tuples (a, b, c, d, e, f) of positive integers satisfy a > b > c > d > e > f and a+f = b+e = c+d = 30 simultaneously?
\binom{14}{3}=364
25 J / 25 S
A timed bomb is equipped with a display showing the time before the explosion in minutes and seconds. It starts counting down with the value 50:00 on the display. A light bulb blinks whenever the displayed number of remaining minutes is equal to the displayed number of remaining seconds (e.g. 15:15) or when the four digits on the display read the same when reversed (e.g. 15:51). We can disable the bomb when the light blinks for the 70th time. What will be the time on the display then?
03:03
26 J / 26 S
Five circles are tangent to each other as indicated in the figure. Find the radius of the smallest circle, if the radius of the big circle is 2 and the two other circles with marked centers are of radius 1.
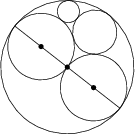
\frac 13
27 J / 27 S
In a casino, some people sat around a large table playing roulette. When Erich left that table carrying his assets of 16\,000 euros away, the average balance of all players decreased by 1\,000 euros. It diminished again by 1\,000 euros, when the two gamblers Bettina and Elfi got into business at that table joining in with 2\,000 euros each. How many players sat around the table while Erich was still gambling?
9
28 J / 28 S
In a cube 7\times7\times7, each two neighboring unit cubes are separated by a partition. We want to remove some of the partitions so that each unit cube will become connected with at least one of the outer unit cubes. What is the minimum number of partitions to be removed?
125
29 J / 29 S
It is known that 20{\ast}{\ast}{\ast} 16 is a 7-digit square of an integer. What are the three missing digits?
909
30 J / 30 S
Triangle ABC with AB = AC = 5\,\text{m} and BC = 6\,\text{m} is partially filled with water. When the triangle lies on the side BC, the surface of the water is 3\,\text{m} above the side. What is the height in meters of the area filled with water when the triangle lies on the side AB?
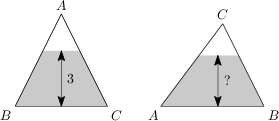
18/5
31 J / 31 S
There are six boxes numbered 1 to 6 and 17 peaches somehow distributed in them. The only move we are allowed to do is the following: If there are exactly n peaches in the n-th box, we eat one of them and add the remaining n-1 peaches to the boxes 1 to n-1, one to each box. What is the distribution of the peaches provided that we can eat all the peaches?
1,1,3,2,4,6
32 J / 32 S
A simple aerial lift with fixed two-person chairs operates on a mountain. 74 people are planning to travel upwards, whereas 26 passengers are waiting at the upper station. Exactly at noon, the lift starts working and a pair of people gets on the lift on both its stations; the rest of the passengers is then loaded continuously. At 12:16, the leading chair going upwards meets the last occupied chair going downwards, and at 12:22, the leading chair going downwards meets the last occupied chair going upwards. The distance between each two chairs on the rope is the same, the lift maintains constant speed, and all the passengers travel in pairs. How long does it take from the lower station to the upper (in minutes)?
26
33 J / 33 S
Let ABCD be a rhombus and M, N points on the segments AB, BC different from A, B, C such that DMN is an equilateral triangle and AD = MD. Find \angle ABC (in degrees).
100^\circ
34 J / 34 S
In how many ways is it possible to color the cells of a 2 \times 7 table with green and yellow in such a way that neither green nor yellow L-trimino appears in the table?
Note: L-trimino is the following (possibly rotated) shape:

130
35 J / 35 S
Michael is a keen diamond collector, but so far he owns less than 200 diamonds. He divided all his diamonds into several (at least two) piles in such a way that
- each two piles consist of different number of diamonds,
- none of these piles consists of exactly two diamonds,
- for each of these piles it holds that whenever it is divided into two smaller piles, at least one of these new piles has the same size as some previously existing one.
What is the greatest number of diamonds Michael can possess?
Note: A pile consists of a non-zero number of diamonds.
196
36 J / 36 S
Recall that in the game of rock-paper-scissors we have three shapes: R – rock, P – paper and S – scissors such that S>P, P>R, R>S and R=R, P=P, S=S, where A>B means ’A beats B’ and A=B means ’when A is played against B, the game ends in a tie’. A tournament in Two Handed Rock-Paper-Scissors Without Repetition between players P_1 and P_2 consists of 9 games. In every game each player chooses a pair (\ell_i,r_i) where \ell_i and r_i stand for shapes played by the left and right hand, respectively, of the player P_i. During the whole tournament each player must choose every possible pair exactly once. In a single game we distribute 4 points in the following way: the winner on each pair of playing hands (left/right) receives 2 points and the loser receives 0 points or both player receive 1 point if there is a tie in a pair of hands. Suppose that players are choosing their moves at random. What is the probability that each of the 9 games in the tournament ends in a tie (i.e. with score 2:2)?
3!^3/9!=1/1680
37 J / 37 S
The net of a solid consists of eight regular triangles and six squares, as shown in the picture:

Assuming that the length of each edge is 1\,\text{km}, what is the volume of the solid (in \text{km}^3)?
\frac 53 \sqrt 2
38 J / 38 S
Find the only three-digit prime factor of 999\,999\,995\,904.
601
39 J / 39 S
Thirteen bees: one little bee and twelve large bees are living on a 37-cell honeycomb. Each large bee occupies 3 pairwise adjacent cells and the little bee occupies exactly 1 cell (see the picture). In how many ways can the honeycomb be divided into 13 non-overlapping sectors so that all thirteen bees can be accommodated in accordance with the given restrictions?
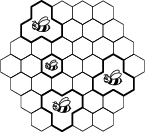
20
40 J / 40 S
Equilateral triangle ABC is inscribed in circle \omega. Point X is on the (shorter) arc BC of \omega and T is the intersection of AB and CX. If AX = 5 and TX = 3, find BX.
15/8
41 J / 41 S
Let ABC be an equilateral triangle. An interior point P of ABC is said to be shining if we can find exactly 27 rays emanating from P intersecting the sides of the triangle ABC such that the triangle is divided by these rays into 27 smaller triangles of equal area. Determine the number of shining points in ABC.
\binom{26}{2} = 325
42 J / 42 S
How many positive divisors of 2016^2 less than 2016 are not divisors of 2016?
47
43 J / 43 S
Let Compute Z_1 + Z_2 + \dots + Z_{2016}.
\frac12 (4033\sqrt{4033} - 1)
44 J / 44 S
We construct a sequence of integers a_0, a_1, a_2 \dots in the following way: If a_i is divisible by three, let a_{i+1}=a_i /3; otherwise let a_{i+1}=a_i +1. For how many different positive integers a_0 does the sequence reach the value 1 for the first time in exactly eleven steps (i.e. a_{11} = 1, but a_0, a_1, \dots, a_{10} \neq 1)?
423
45 J / 45 S
Let ABCD, AEFG, and EDHI be rectangles with centers K, L, J, respectively. Assume further that A, D, E are inner points of line segments HI, FG, BC, respectively, and \angle AED = 53^\circ. Determine the size of \angle JKL (in degrees).
74^\circ
46 J / 46 S
James has picked several (not necessarily distinct) integers from the set \{-1, 0, 1, 2\} in such a way that their sum equals 19 and the sum of their squares is 99. What is the greatest possible value of the sum of the cubes of James’ numbers?
133
47 J / 47 S
Find the largest 9-digit number with the following properties:
- all of its digits are different;
- for each k=1,2,\ldots,9, when the k-th digit is crossed out the resulting 8-digit number is divisible by k.
876\,513\,240
48 J / 48 S
Point P lies inside a rectangle ABCD with AB=12. Each of triangles ABP, BCP, DAP has its perimeter equal to its area. What is the perimeter of triangle CDP?
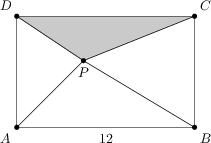
25
49 J / 49 S
The pair of integers (0,0) is written on a blackboard. In each step, we replace it in this way: If there is a pair (a,b), we replace it with (a+b+c,b+c), where either c=247 or c=-118 (we may choose the number c in each step). Find the smallest (non-zero) number of steps after which the pair (0,b) for some b appears on the blackboard.
145
50 J / 50 S
A zigzag consists of two parallel rays of opposite directions with the initial points joined with a segment. What is the maximum number of regions the plane can be divided into using ten zigzags?
416
51 J / 51 S
Each face of a tetrahedron is a triangle with sides 1, \sqrt 2, and c and the circumradius of the tetrahedron is 5/6. Find c.
\sqrt{23}/3
52 J / 52 S
For a big welcome party butler James has lined up 2016 cocktail glasses in a row, each containing delicious cherry cocktail. To finish things up, his task is to cover one of the glasses with a silver lid, to put a statue on top of the lid and to distribute an odd number of cherries into the uncovered glasses, at most one cherry per glass. How many possible arrangements of cherries and the lid are there if there have to be more cherries on the right-hand side of the lid than on its left-hand side?
2016\cdot 2^{2013}
53 J / 53 S
We are given a wooden cube with its surface painted green. There are 33 different planes, each located between some two opposite faces of the cube and parallel to them, which dissect the cube into small cuboidal blocks. Given that the number of blocks with at least one green face equals the number of blocks with no green faces, determine the total number of blocks into which the cube is dissected.
1260 or 1344
54 J / 54 S
Given a positive integer n, let p(n) be the product of non-zero digits of n. Find the largest prime divisor of the number p(1)+\dots+p(999).
103
55 J / 55 S
Let (a_n)_{n=1}^\infty be a strictly increasing sequence of positive integers such that 9\mid a_{3k-2}, 14\mid a_{3k-1}, and 19\mid a_{3k} for all positive integers k. Find the smallest possible value of a_{2016}.
14478
56 J / 56 S
Let P be a point inside triangle ABC. Points D, E, F lie on the segments BC, CA, AB, respectively, such that the lines AD, BE, CF intersect in P. Given that PA=6, PB=9, PD=6, PE=3, and CF=20, find the area of triangle ABC.
108
57 J / 57 S
Find the last two digits before the decimal point of the number (7 + \sqrt{44})^{2016}.
05